Potentiel vecteur créé par un dipôle magnétostatique [Les courants stationnaires : champ et potentiel vecteur magnétostatiques]

Potentiel vecteur créé par un dipôle magnétostatique

On appelle dipôle magnétostatique une distribution de courants dont les dimensions sont faibles par rapport aux distances auxquelles on se place pour étudier ses effets.

Soit un circuit filiforme \(\mathsf{C}\) parcouru par un courant continu d'intensité \(\mathsf{I}\).

Le potentiel vecteur créé par cette distribution de courant en un point \(\mathsf{M}\), a pour expression :
\(\vec A(M) = \frac{\mu_0}{4\pi} \oint_{\mathcal{C}} I \frac{\overrightarrow{\mathrm{d}l}(P)}{PM}\)
Soit un champ uniforme quelconque de vecteur unitaire \(\vec u\).
Le produit scalaire \(\vec A(M) . \vec u\) s'écrit :
\(\vec A(M) . \vec u = \frac{\mu_0I}{4\pi} \oint_{\mathcal{C}} . \frac{\vec u .\mathrm{ }\overrightarrow{\mathrm{d}l}(P)} {PM}\)
En appliquant la formule de Stokes (voir rotationnel^[1]) on a :
\(\vec A(M) . \vec u = \frac{\mu_0I}{4\pi} \oint_{\mathcal{C}} . \frac{\vec u}{PM} .\mathrm{ }\overrightarrow{\mathrm{d}l}(P) = \frac{\mu_0I}{4\pi}\iint_{\mathfrak{S}}(\stackrel{\hookrightarrow}{\mathrm{rot}} \frac{\vec u}{PM}) . \stackrel{\hookrightarrow}{\mathrm{d}\mathfrak{S}}\)
\(\mathfrak{S}\) étant une surface ouverte s'appuyant sur le circuit \(\mathsf{C}\), l'orientation de \(\mathfrak{S}\) est fixée par le sens de circulation du courant dans le circuit \(\mathsf{C}\).
Dans le calcul de l'intégrale précisons que \(\mathsf{P}\) est le point courant et \(\mathsf{M}\) le point fixe.

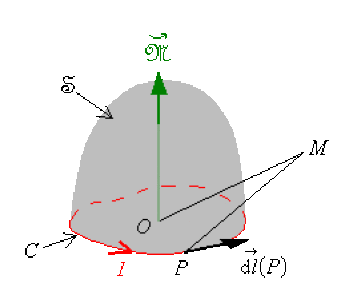
Ainsi nous explicitons \(\stackrel{\hookrightarrow}{\mathrm{rot}} \frac{\vec u}{PM}\) par rapport aux coordonnées de \(\mathsf{P}\) et pour lever toute ambiguïté nous le précisons en indice
\(\stackrel{\hookrightarrow}{\mathrm{rot}_p} \vec u \frac{1}{PM} = \frac{1}{PM} \stackrel{\hookrightarrow}{\mathrm{rot}_p}\vec u + \overrightarrow{\mathrm{grad}}_{p} \frac{1}{PM} \wedge \vec u = \overrightarrow{\mathrm{grad}}_{p} \frac{1}{PM}\wedge \vec u~,\)
car \(\stackrel{\hookrightarrow}{\mathrm{rot}_p} \vec u = \stackrel{\hookrightarrow}{0}\).
Comme \(\overrightarrow{\mathrm{grad}}_{p} \frac{1}{PM} = \frac{\overrightarrow{PM}}{PM^3}~,\)
on a : \(\stackrel{\hookrightarrow}{\mathrm{rot}_p} \frac{\vec u}{PM} = \frac{\overrightarrow{PM}}{PM^3}\wedge \vec u\)
On peut alors écrire : \(\displaystyle{\vec A(M) . \vec u = \frac{\mu_0I}{4\pi} \iint_{\mathfrak{S}}\Big[\Big(\frac{\overrightarrow{PM}}{PM^3}\wedge \vec u\Big) . \stackrel{\hookrightarrow}{\mathrm{d}\mathfrak{S}}\Big]}\)
Le produit mixte^[2] sous le signe somme peut s'expliciter d'une manière différente :
\(\displaystyle{\vec A(M) . \vec u = \frac{\mu_0I}{4\pi} \iint_{\mathfrak{S}}\Big[\Big(\frac{\overrightarrow{PM}}{PM^3}\wedge \vec u \Big) . \stackrel{\hookrightarrow}{\mathrm{d}\mathfrak{S}}\Big] = \frac{\mu_0I}{4\pi} \iint_{\mathfrak{S}}\Big[\Big(\stackrel{\hookrightarrow} {\mathrm{d}\mathfrak{S}} \wedge \frac{\overrightarrow{PM}}{PM^3}\Big) . \vec u\Big]}\)
Cette relation étant valable quelque soit \(\vec u\) (démonstration^[3]), on a :
\(\displaystyle{\vec A(M) . \vec u = \frac{\mu_0I}{4\pi} \Big[\iint_{\mathfrak{S}} \stackrel{\hookrightarrow}{\mathrm{d}\mathfrak{S}} \wedge \frac{\overrightarrow{PM}}{PM^3}\Big] . \vec u}\)
Soit : \(\vec A(M) = \frac{\mu_0I}{4\pi} \iint_{\mathfrak{S}} \stackrel{\hookrightarrow}{\mathrm{d}\mathfrak{S}} \wedge \frac{\overrightarrow{PM}}{PM^3}\)
Compte tenu de la définition du dipôle magnétostatique, les propriétés du circuit \(\mathsf{C}\) sont étudiées à grande distance et on peut remplacer \(\overrightarrow{PM}\) par \(\overrightarrow{OM}\), \(\mathsf{O}\) n'étant pas n'importe quel point mais "un point moyen du circuit". On a ainsi :
\(\vec A(M) = \frac{\mu_0I}{4\pi} \iint_{\mathfrak{S}} \stackrel{\hookrightarrow}{\mathrm{d}\mathfrak{S}} \wedge \frac{\overrightarrow{OM}}{OM^3}\) que l'on peut aussi écrire, puisque \(\mathsf{O}\) et \(\mathsf{M}\) sont fixés
\(\displaystyle{\vec A(M) = \frac{\mu_0}{4\pi}\Big(I \iint_{\mathfrak{S}} \stackrel{\hookrightarrow}{\mathrm{d}\mathfrak{S}}\Big) \wedge \frac{\overrightarrow{OM}}{OM^3}}\)

Notes

Rotationnel
- On appelle rotationnel au point \(\mathsf{M}\) du champ vectoriel \(\vec B(M)\), le vecteur que l'on note \(\stackrel{\hookrightarrow}{\mathrm{rot}}\vec B(M)\) défini par la formule de Stokes :
  \(\oint_{\mathcal{C}}\stackrel{\hookrightarrow}{B}(M_{\mathcal{C}}) = \iint_{\mathfrak{S}}\stackrel{\hookrightarrow}{\mathrm{rot}}\vec B(M_{\mathfrak{S}}) . \stackrel{\hookrightarrow}{\mathrm{d}\mathfrak{S}}(M_{\mathfrak{S}})\)
  \(M_{\mathcal{C}}\) étant un point du contour fermé orienté \(\textcircled{C}\) et \(M_{\mathfrak{S}}\) un point de la surface ouverte \((\mathfrak{S})\) s'appuyant sur \(\textcircled{C}\).
- La circulation du champ vectoriel \(\vec B(M)\) le long d'un contour fermé quelconque \(\textcircled{C}\) est égale au flux du champ vectoriel \(\stackrel{\hookrightarrow}{\mathrm{rot}}\vec B(M)\) à travers toute surface ouverte \((\mathfrak{S})\) s'appuyant sur \(\textcircled{C}\).
- L'opérateur rotationnel fait passer d'un champ vectoriel \(\vec B(M)\) à un autre champ vectoriel \(\stackrel{\hookrightarrow}{\mathrm{rot}}\vec B(M)\) ; il peut donner des informations sur le caractère "tourbillonnaire" de \(\vec B(M)\).
- Expressions du rotationnel de \(\vec B(M)\) dans différents systèmes de repérage
  Repérage cartésien :
  \(\displaystyle{\stackrel{\hookrightarrow}{\mathrm{rot}}\vec B(M) = \Big(\frac{∂B_z}{∂y} - \frac{∂B_y}{∂z}\Big) \vec e_x + \Big(\frac{∂B_x}{∂z} - \frac{∂B_z}{∂x}\Big) \vec e_y + \Big(\frac{∂B_y}{∂x} - \frac{∂B_x}{∂y}\Big) \vec e_z}\)
  Repérage cylindrique :
  \(\displaystyle{\stackrel{\hookrightarrow}{\mathrm{rot}}\vec B(M) = \frac{1}{\rho}\Big(\frac{∂B_z}{∂\phi} - \frac{∂(\rho B_{\phi})}{∂z}\Big) \vec e_{\rho} + \Big(\frac{∂B_{\rho}}{∂z} - \frac{∂B_z}{∂{\rho}}\Big) \vec e_{\phi} + \frac{1}{\rho}\Big(\frac{∂(\rho B_{\phi})}{∂\rho} - \frac{∂B_{\rho}}{∂\phi}\Big) \vec e_z}\)
  Repérage sphérique :
  \(\displaystyle{\stackrel{\hookrightarrow}{\mathrm{rot}}\vec B(M) = \frac{1}{r\sin\theta}\Big(\frac{∂B_{\phi}\sin\theta}{∂\theta} - \frac{∂B_{\theta}}{∂\phi} \Big)\vec e_r + \frac{1}{r}\Big(\frac{1}{\sin\theta}\frac{∂B_r}{∂\phi} - \frac{∂(B_{\phi})}{∂r}\Big) \vec e_{\theta} + \frac{1}{r}\Big(\frac{∂(B_{\theta})}{∂r} - \frac{∂B_r}{∂\theta}\Big) \vec e_\phi}\)
Produit mixte
Étant donné trois vecteurs \(\vec V_1\), \(\vec V_2\) et \(\vec V_3\), leur produit mixte est un scalaire \(\mathsf{p}\) tel que \(p = (\vec V_1 \wedge \vec V_2).\vec V_3\). Ce produit est invariant si on effectue une permutation circulaire sur les trois vecteurs. Ainsi : \(p = (\vec V_1 \wedge \vec V_2).\vec V_3 = (\vec V_3 \wedge \vec V_1).\vec V_2 = (\vec V_2 \wedge \vec V_3) . \vec V_1\)
\(\mathsf{P}\) représente le volume du parallélépipède construit sur \(\vec V_1\), \(\vec V_2\) et \(\vec V_3\). En effet, en posant\( \vec P = (\vec V_1 \wedge \vec V_2)\), on a :
\(p = (\vec V_1 \wedge \vec V_2).\vec V_3 = \vec P .\vec V_3 = \parallel \vec P\parallel \parallel\vec V_3\parallel \cos \alpha = \parallel\vec P \parallel . h\)
Appliquée au champ magnétique la permutation donne :
\((\vec v \wedge \vec B) . \vec v = ( \vec v \wedge \vec v).\vec B = \vec0\) car \(\parallel \vec v \wedge \vec v\parallel = v^2 \sin(\vec v,\vec v) = v^2\sin(0) = 0\)
Démonstration
Considérons deux vecteurs \(\vec V_1\) et \(\vec V_2\) et un champ uniforme de vecteurs unitaires \(\vec u\).
On peut écrire : \((\vec V_1 + \vec V_2) . \vec u = (\vec V_1 . \vec u + \vec V_2 . \vec u)\) et généraliser pour \(\mathsf{n}\) vecteurs,
ce qui donne :
\(\left(\sum_{i=1}^n \vec{V_i}\right).\vec{u}=\left\{\sum_{i=1}^n(\vec{V_i}.\vec{u})\right\}\)
Si le nombre de vecteurs devient infini, la somme \(\left\{\sum_{i=1}^n\right\}\) est alors remplacée par une intégrale soit, pour le cas qui nous concerne, en posant \(K = \frac{\mu_0I}{4\pi}\) et \(\overrightarrow{\mathrm{d}V} = \stackrel{\hookrightarrow}{\mathrm{d}\mathfrak{S}} \wedge \frac{\overrightarrow{PM}}{PM^3}\) :
\(K(\iint_{\mathfrak{S}} \overrightarrow{\mathrm{d}V}) . \vec u = K \iint_{\mathfrak{S}}(\overrightarrow{\mathrm{d}V} . \vec u)\)
Comme \(\vec A . \vec u = K\iint_{\mathfrak{S}}(\overrightarrow{\mathrm{d}V}. \vec u)\) on a alors
\(\vec A . \vec u = K\iint_{\mathfrak{S}}(\overrightarrow{\mathrm{d}V}. \vec u) = K(\iint_{\mathfrak{S}} \overrightarrow{\mathrm{d}V}) . \vec u\)
ce qui donne : \(\vec A = K(\iint_{\mathfrak{S}} \overrightarrow{\mathrm{d}V})\) soit \(\vec A(M) = \frac{\mu_0I}{4\pi} \iint_{\mathfrak{S}} \stackrel{\hookrightarrow}{\mathrm{d}\mathfrak{S}} \wedge \frac{\overrightarrow{PM}}{PM^3}\).