Force de Laplace : fil plongé dans un champ uniforme
Partie
Question
Soit un fil rectiligne, parcouru par un courant d'intensité \(I\), plongé dans un champ magnétostatique extérieur uniforme \(\vec B_{\mathrm{ext}}\).

Déterminer la direction et le sens de la force de Laplace élémentaire \(\vec{\mathrm{d} F}\) qui s'exerce sur l'élément de courant \(\vec{\mathrm{d} l}\) dans les cas suivants (\(B_{\mathrm{ext}} > 0\)) :
\(\vec B_{\mathrm{ext}} = B_{\mathrm{ext}} . \vec{e_x}\)
\(\vec B_{\mathrm{ext}} = - B_{\mathrm{ext}} . \vec{e_x}\)
\(\vec B_{\mathrm{ext}} = B_{\mathrm{ext}} . \vec{e_z}\)
\(\vec B_{\mathrm{ext}} = B_{\mathrm{ext}} . \vec{e_y}\)
\(\vec B_{\mathrm{ext}} = B_x . \vec{e_x} + B_z . \vec{e_z} \quad\) (\(B_x \mathrm{ et } B_z > 0\))
Aide simple
D'après la loi de Laplace la force élémentaire \(\vec{\mathrm{d} F}\) dépend du produit vectoriel \(\vec{\mathrm{d} l} \wedge \vec B\).
Rappel de cours
Le produit scalaire :
\(\vec A.\vec B=\left(\begin{array}{c} A_1\\A_2\\A_3\end{array} \right)_{\mathcal{B}} . \left(\begin{array}{c} B_1\\B_2\\B_3\end{array} \right)_{\mathcal{B}} =A_1B_1+A_2B_2+A_3B_3\)
Le produit vectoriel :
\(\vec A\wedge\vec B=\left(\begin{array}{c} A_1\\A_2\\A_3\end{array} \right)_{\mathcal{B}} \wedge\left(\begin{array}{c} B_1\\B_2\\B_3\end{array} \right)_{\mathcal{B}} =\left(\begin{array}{c} A_2B_3-A_3B_2\\A_3B_1-A_1B_3\\A_1B_2-A_2B_1\end{array} \right)_{\mathcal{B}}\)
Les opérateurs vectoriels :
Système de repérage cartésien \(\mathcal{B}_{\mathrm{cart.}}(\vec{e_x}, \vec{e_y}, \vec{e_z})\)
gradient : \(\vec{\mathrm{grad}}U\) | divergence : \(\mathrm{div}\vec A\) | rotationnel : \(\vec{\mathrm{rot}}\vec A\) |
\(\left(\begin{array}{c} \displaystyle{ \frac{\partial U}{\partial x} } \\ \displaystyle{ \frac{\partial U}{\partial y} } \\ \displaystyle{ \frac{\partial U}{\partial z} } \end{array} \right)_{\mathcal{B}_{\mathrm{cart.}}}\) | \(\displaystyle{ \frac{\partial A_x}{\partial x}+\frac{\partial A_y}{\partial y}+\frac{\partial A_z}{\partial z} }\) | \(\left(\begin{array}{c} \displaystyle{ \frac{\partial A_z}{\partial y}-\frac{\partial A_y}{\partial z} } \\ \displaystyle{ \frac{\partial A_x}{\partial z}-\frac{\partial A_z}{\partial x} } \\ \displaystyle{ \frac{\partial A_y}{\partial x}-\frac{\partial A_x}{\partial y} }\end{array} \right)_{\mathcal{B}_{\mathrm{cart.}}}\) |
Système de repérage cylindrique \(\mathcal B_{\mathrm{cyl.}}(\vec{e_r},\vec{e_{\theta}},\vec{e_z})\)
gradient : \(\vec{\mathrm{grad}}U\) | divergence : \(\mathrm{div}\vec A\) | rotationnel : \(\vec{\mathrm{rot}}\vec A\) |
\(\left(\begin{array}{c} \displaystyle{ \frac{\partial U}{\partial r} } \\ \displaystyle{ \frac{1}{r} \frac{\partial U}{\partial \theta} }\\ \displaystyle{ \frac{\partial U}{\partial z} } \end{array} \right)_{\mathcal{B}_{\mathrm{cyl.}}}\) | \(\displaystyle{ \frac{1}{r} \frac{\partial (r . A_r) }{\partial r} + \frac{1}{r} \frac{\partial A_{\theta}}{\partial \theta}+\frac{\partial A_z}{\partial z} }\) | \(\left(\begin{array}{c} \displaystyle{ \frac{1}{r} \frac{\partial A_z}{\partial \theta}-\frac{\partial A_ \theta}{\partial z} } \\ \displaystyle{ \frac{\partial A_r}{\partial z}-\frac{\partial A_z}{\partial r} } \\ \displaystyle{ \frac{1}{r} \left( \frac{\partial (r. A_{\theta})}{\partial r}-\frac{\partial A_r}{\partial \theta} \right)} \end{array} \right)_{\mathcal{B}_{\mathrm{cyl.}}}\) |
Nom de l'outil | Comment s'énonce-t-il ? | Quand l'utiliser ? |
Théorème d'Ampère | \(\displaystyle{ \oint_{\mathcal C}\vec B.\vec{\mathrm{d}l}=\mu_0\sum I }\) | Pour calculer \(\vec B\) si la géométrie du problème permet un calcul simple de la circulation de \(\vec B\). |
Loi de Biot et Savart | \(\displaystyle{ \vec B(M)=\frac{\mu_0}{4\pi}\int_{\mathcal D}\vec{\mathrm{d} \mathcal C}(P)\wedge\frac{\vec{PM}}{PM^3} }\) | Pour calculer \(\vec B\) si la géométrie de la distribution ne permet pas une application simple du théorème d'Ampère. |
Relation champ magnétostatique/ potentiel vecteur | \(\vec{B}(M)=\vec{\mathrm{rot}}\vec A(M)\) | Pour calculer \(\vec B\) si \(\vec A\) est connu. |
Définition de la force de Laplace | \(\vec{F_m}=\displaystyle{ \int_{\mathcal D}\vec{\mathrm{d} \mathcal C}(P)\wedge\vec B_{\mathrm{ext}}(P) }\) | Pour calculer la force qui s'exerce sur une distribution \(\mathcal D\) soumise à un champ magnétostatique extérieur \(\vec B_{\mathrm{ext}}\) |
Théorème de Maxwell | \(W_{2\leftarrow1}=I . \Phi_c\) | Pour calculer directement le torseur des forces qui agissent sur un circuit. |
Définition du potentiel vecteur | \(\displaystyle{ \vec A(M)=\int_{\mathcal D}\frac{\mu_0}{4\pi}\frac{\vec{\mathrm{d}\mathcal C}(P)}{PM} }\) | Pour calculer \(\vec A\) si la distribution a un haut degré de symétrie. |
Solution détaillée
La force de Laplace élémentaire est donnée par \(\vec{\mathrm{d} F} = I \vec{\mathrm{d} l} \wedge \vec B\). Sa direction et son sens peuvent être déterminés en effectuant le produit vectoriel dans le système de repérage cartésien proposé, avec :
\(\vec{\mathrm{d} l} = \left( \begin{array}{c} 0 \\ 0 \\ \mathrm{d} z \end{array} \right)\)
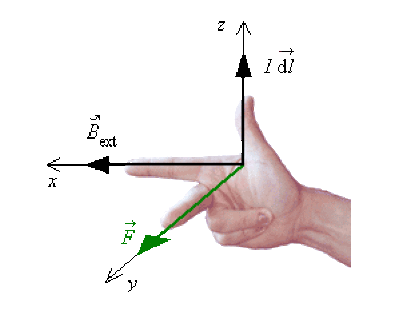
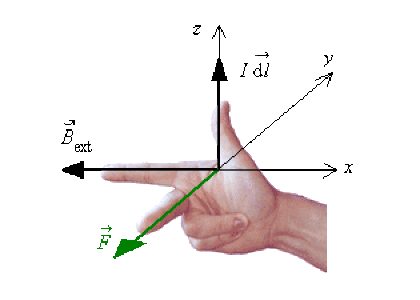
On peut également, dans le cas où \(\vec{\mathrm{d} l}\) et \(\vec B\) sont perpendiculaires, utiliser la règle des 3 doigts.
On obtient :
si \(\vec B_{\mathrm{ext}} = B_{\mathrm{ext}} . \vec{e_x}\) ,
\(\vec{\mathrm{d} F} = I . \mathrm{d} l . B_{\mathrm{ext}} . \vec{e_y}\)
si \(\vec B_{\mathrm{ext}} = - B_{\mathrm{ext}} . \vec{e_x}\) ,
\(\vec{\mathrm{d} F} = - I . \mathrm{d} l . B_{\mathrm{ext}} . \vec{e_y}\)
si \(\vec B_{\mathrm{ext}} = B_{\mathrm{ext}} . \vec{e_z}\) ,
\(\vec{\mathrm{d}F} = 0\) (dans ce cas-ci \(\vec{\mathrm{d} l}\) et \(\vec B\) sont colinéaires, d'où un produit vectoriel nul)
si \(\vec B_{\mathrm{ext}} = B_{\mathrm{ext}} . \vec{e_y}\) ,
\(\vec{\mathrm{d} F} = - I . \mathrm{d} l . B_{\mathrm{ext}} . \vec{e_x}\)

si \(\vec B_{\mathrm{ext}} = B_x . \vec{e_x} + B_z . \vec{e_z}\) ,
\(\vec{\mathrm{d} F} = I \vec{\mathrm{d} l} \wedge \left( B_x . \vec{e_x} + B_z . \vec{e_z} \right) = I . \mathrm{d} l . B_x . \vec{e_y} + 0 = I . \mathrm{d} l . B_x . \vec{e_y}\)