Nombres complexes

Soit \(\vec{P}\) le plan vectoriel euclidien rapporté à la base orthonormée \(\left(\overrightarrow{e_{1}}, \overrightarrow{e_{2}}\right)\) et soit \(P\) le plan affine euclidien rapporté au repère orthonormé \(\left(O, \overrightarrow{e_{1}}, \overrightarrow{e_{2}}\right)\)

Les applications, dans lesquelles \((a,b) \in R^{2}\)

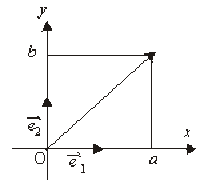
\(\begin{array}{ll}\vec{f} \quad \mathbb{C} \to \vec{P} \\ \underline{z} = a + j b \mapsto \overrightarrow{V} = a~ \overrightarrow{e_{1}} + b~ \overrightarrow{e_{2}} \end{array} \) et \(\begin{array}{ll}f \quad \mathbb{C} \to P \\ \underline{z} = a + j b \mapsto M~ (\textrm{avec }\overrightarrow{OM} = a ~\overrightarrow{e_{1}} + b ~\overrightarrow{e_{2}}) \end{array}\) sont des bijections

On dit que \(\overrightarrow{V} =a~ \overrightarrow{e_{1}} + b~ \overrightarrow{e_{2}}\) est le vecteur image du complexe \(\underline{z} = a + j b\) dans le plan vectoriel euclidien \(\vec{P}\) rapporté à la base orthonormée \(\left(\overrightarrow{e_{1}}, \overrightarrow{e_{2}}\right)\)

De même \(M\) avec \(\overrightarrow{OM} =a~ \overrightarrow{e_{1}} + b~ \overrightarrow{e_{2}}\) est le point image du complexe \(\underline{z} = a + j b\) dans le plan affine euclidien \(P\) rapporté au repère orthonormé \(\left(O, \overrightarrow{e_{1}}, \overrightarrow{e_{2}}\right)\)

Réciproquement : Pour tout \(\underline{z} = a + j b \in \mathbb{C}\), on dit que le complexe \(\underline{z} = a + j b\) est :

• l'affixe du vecteur \(\overrightarrow{V}\) par l'application \(\overrightarrow{f^{-1}}\left(\overrightarrow{V}\right)\) pour tout \(\overrightarrow{V} \in \vec{P}\)

• l'affixe du point \(M\) par l'application \(f^{-1} (M)\) pour tout \(M \in P\)

Le plan \(P\) est appelé le "plan complexe".

L'axe des abscisses représente l'ensemble des réels \(\mathbb{R}\) .

L'axe des ordonnées représente l'ensemble des imaginaires purs \(j \mathbb{R}\) .