Fonction Argument cosinus hyperbolique de x
Définition :
L'application \(\textrm{ch}\) : \([0,+\infty] \to [1,+\infty]\) continue et strictement croissante admet une fonction réciproque notée \(\textrm{Argch}\) : \([1,+\infty] \to [0,+\infty]\)
\(y = \textrm{Argch } x \Leftrightarrow x = \textrm{ch }y\)
\(x \in [1,+\infty[ \textrm{ et } y \in [0,+\infty[\)
\(\begin{array}{llr} \textrm{sh } (\textrm{Argch }x) = \sqrt{x^{2} - 1} & \forall x \in [1,+\infty[ \\ \textrm{ch } (\textrm{Argch }x) = 1& \forall x \in [1,+\infty[ \\\textrm{th } (\textrm{Argch }x) = \frac{\sqrt{x^{2}-1}}{x} \qquad& \forall x \in [1,+\infty[ \\ \textrm{coth } (\textrm{Argch }x) = \frac{x}{\sqrt{x^{2}-1}} \qquad& \forall x \in [1,+\infty[\end{array}\)
fonction ni paire, ni impaire, d'où \(D_{e} =D_{f} = [1, + \infty [\)
fonction dérivable sur \(]1,+\infty[\) et :
\(\textrm{Argch'}x = \frac{1}{\textrm{ch}'(\textrm{Argch }x)} = \frac{1}{\textrm{sh}(\textrm{Argch }x)} = \frac{1}{\sqrt{x^{2} - 1}}>0\)
donc fonction croissante sur \(D_{e}\).
tableau de variation :
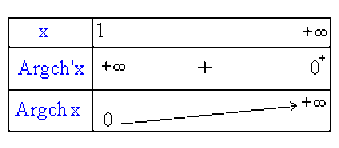
Représentation graphique de \(\textrm{Argch }x\)
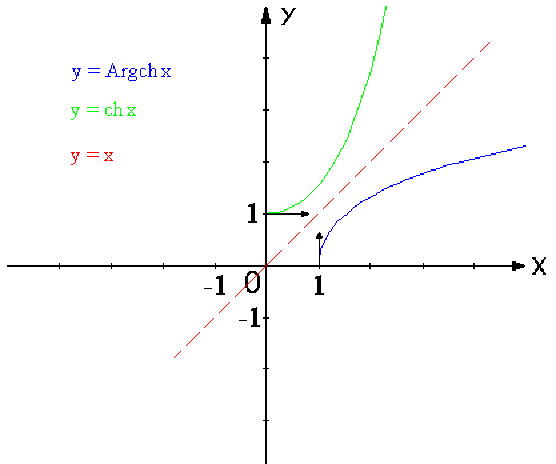
Expression logarithmique de \(\textrm{Argch }x\)
\(\forall x \in [1,+\infty[ \qquad \textrm{Argch }x = \ln \left(x + \sqrt{x^{2} - 1}\right)\)
Démonstration :
\(\forall (x, y) \in [1,+\infty[ \times [0,+\infty[,\)
\(y = \textrm{Argch }x \Leftrightarrow x = \textrm{ch }y \Leftrightarrow \textrm{sh }y = + \sqrt{\textrm{ch}^{2}y - 1} = \sqrt{x^{2} - 1}\)
et comme
\(e^{y} = \textrm{ch }y + \textrm{sh }y = x + \sqrt{x^{2} - 1}\)
\(y = \ln \left(x+\sqrt{x^{2} - 1}\right)\)
Remarque :
Pour \(y \in [0,+\infty[\), \(\textrm{sh }y\) est positif, d'où la solution retenue \(\textrm{sh }y = (\textrm{ch}^{2}y - 1)^{ 1/2}\) .
On retrouve la fonction dérivée :
\(\left[\ln\left(x+ \sqrt{x^{2}-1}\right)\right]' = \frac{1+\frac{x}{\sqrt{x^{2} - 1}}}{x + \sqrt{x^{2} - 1}} = \frac{1}{\sqrt{x^{2} - 1}} = \textrm{Argch}' x\)