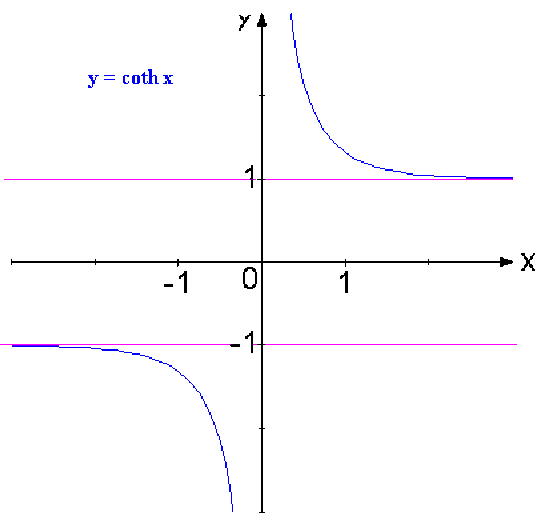Fonction cotangente hyperbolique de x : coth x
La fonction cotangente hyperbolique, notée \(\textrm{coth}\) , est :
définie sur \(\mathbb{R}^{\ast}\) :
\(\textrm{coth}x = \frac{\textrm{ch}x}{\textrm{sh}x} = \frac{e^{x} + e^{-x}}{e^{x} - e^{-x}} = \frac{e^{2x} + 1}{e^{2x} - 1} = \frac{1+e^{-2x}}{1- e^{-2x}}\)
impaire :
\(\textrm{coth} (-x) = \frac{\textrm{ch}(-x)}{\textrm{sh} (-x)} = - \frac{\textrm{ch}x}{\textrm{sh}x} = - \textrm{coth}x\)
Le domaine d'étude se réduit à \(D_{e} = ]0, +\infty [\) . Le graphe de la fonction cotangente hyperbolique, admet l'origine \(O\) comme centre de symétrie.
Aux bornes de \(D_{e}\), nous avons :
\(\displaystyle{\lim_{x \to 0^{+}} \textrm{coth }x \approx \lim_{x \to 0^{+}} \frac{\textrm{ch }x}{ \textrm{sh }x} =\frac{1}{0^{+}} = + \infty}\)
La droite d'équation \(x = 0\) (axe des ordonnées) est une asymptote verticale.
\(\displaystyle{\lim_{x \to + \infty} \textrm{coth }x \approx \lim_{x \to +\infty} \frac{e^{2x}}{e^{2x}} = 1}\)
la droite d'équation \(y = 1\) est une asymptote horizontale.
La fonction dérivée \(\textrm{coth'} x = (\textrm{ch }x / \textrm{sh }x )' = (\textrm{sh}^{2}x - \textrm{ch}^{2}x) / \textrm{sh}^{2}x = 1 - \textrm{coth}^{2}x =- 1 / \textrm{sh}^{2}x\) étant négative sur \(\textrm{D}_{e}\), la fonction \(\textrm{coth }x\) est décroissante sur \(\textrm{D}_{e}\) .
Tableau de variation sur \(D_{e}\)
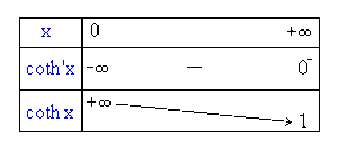
Représentation graphique de \(\textrm{coth }x\)