Division
Division algébrique
\(\begin{array}{ll}\underline{z}=\frac{\underline{z}'}{\underline{z}''} &\Leftrightarrow a+jb=\frac{a'+jb'}{a''+jb''}=\frac{(a'+jb')(a''-jb'')}{(a''+jb'')(a''-jb'')} = \frac{(a'a''+b'b'')+j(a''b'-a'b'')}{a''^{2} + b''^{2}}\\ \\ &\Leftrightarrow \left\{\begin{array}{ll} a= \frac{a'a'' + b'b''}{{a''}^{2}+{b''}^{2}} \\ \\ b= \frac{a''b' - a'b''}{{a''}^{2}+{b''}^{2}} \end{array}\right. \end{array}\)
Division "polaire"
\(\begin{array}{ll}\underline{z} = \frac{\underline{z}'}{\underline{z}''} \Leftrightarrow \rho(\cos \theta + j \sin \theta) & = \frac{\rho'(\cos \theta ' + j \sin \theta')} {\rho''(\cos \theta'' + j \sin \theta'')} = \frac{\rho'}{\rho''}(\cos \theta' + j \sin \theta')(\cos \theta'' - j \sin \theta'')\\ & =\frac{\rho'}{\rho''}\left[\left(\cos \theta'\cos\theta''+\sin\theta'\sin\theta''\right)\right. + \\& \left.j \left(\sin \theta'\cos\theta''-\cos\theta'\sin\theta''\right)\right] \\ & = \frac{\rho'}{\rho''}\left[\cos(\theta' - \theta'') + j \sin(\theta' - \theta'')\right]\end{array}\)
\(\rho e^{j \theta} = \frac{\rho' e^{j \theta'}}{ \rho'' e^{j \theta''}} = \frac{\rho'}{\rho''} e^{j(\theta'-\theta'')}\Leftrightarrow \left\{\begin{array}{ll} \rho = \frac{\rho'}{\rho''} \\ \theta = \theta' - \theta'' \end{array}\right.\)
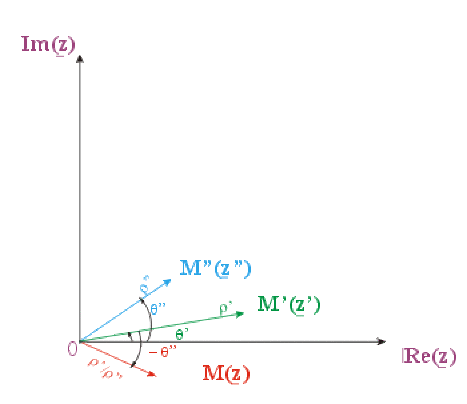
Division "vecteur-image"
Des résultats de la division "polaire", diviser le nombre complexe \(\underline{z}'\), de vecteur-image \(\overrightarrow{OM}'\) par le nombre complexe \(\underline{z}''\) de module \(\rho''\) et d'argument \(\theta''\) consiste à faire subir au "vecteur-image" \(\overrightarrow{OM}'\) une rotation d'angle \(-\theta''\) et une division de son module par \(\rho''\).
Conclusion
De nouveau la forme polaire est préférée dans le cas de la division de deux nombres complexes
Division des modules
Soustraction des arguments
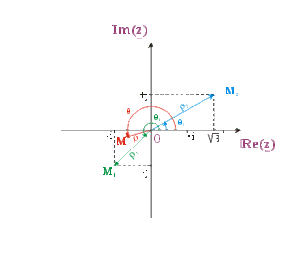
Exemple :
Soient les nombres complexes
\(\underline{z_{1}} = -1-j\) et \(\underline{z_{2}} =\sqrt{3} +j\) (formes algébriques)
\(\underline{z_{1}} = \sqrt{2} \left[\cos\frac{5 \pi}{4} + j \sin \frac{5 \pi}{4}\right]\)
et \(\underline{z_{2}} = 2 \left[\cos\frac{\pi}{6} + j \sin \frac{\pi}{6}\right]\) (formes trigonométriques)
Division "algébrique":
\(\frac{\underline{z}_{1}}{\underline{z}_{2}} = \frac{-1-j}{\sqrt{3}+j} = \frac{-\left(1+j\right)\left(\sqrt{3}-j\right)}{\left(\sqrt{3}+j\right)\left(\sqrt{3}-j\right)} = \frac{-1}{4} \left(\sqrt{3} + 1 + j\left(\sqrt{3} - 1 \right)\right)\)
Division"polaire":
\(\frac{\underline{z}_{1}}{\underline{z}_{2}} = \frac{-1-j}{\sqrt{3}+j} = \frac{-\left(1+j\right)\left(\sqrt{3}-j\right)}{\left(\sqrt{3}+j\right)\left(\sqrt{3}-j\right)} = \frac{-1}{4} \left(\sqrt{3} + 1 + j\left(\sqrt{3} - 1 \right)\right)\)
Division "vecteur-image"